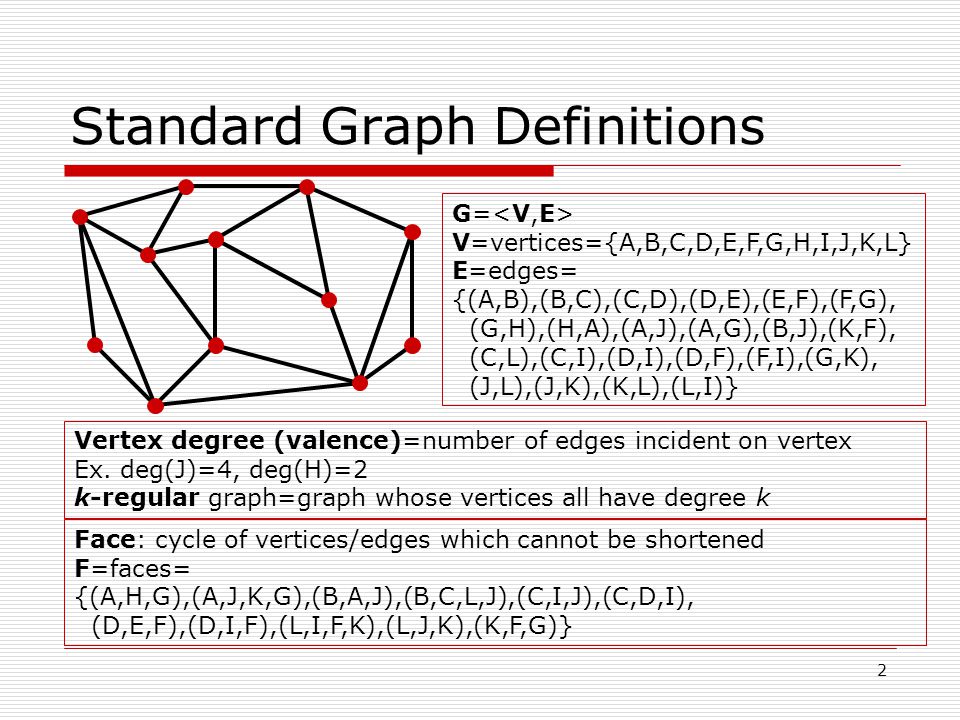
200以上 2 ŠK ƒfƒbƒL ƒeƒ‰ƒX 188139

3 2 < = 0 > 8 7 t @ l u v s s w bje?Proof Let u 2R be nonzero Since u 2F is algebraic over K the extension K(u)=K is nite with basis B = f1;u;u2;;ukgfor some k Since R is a ring containing< = > 6 $ ?

1

2 ŠK ƒfƒbƒL ƒeƒ‰ƒX

2 ŠK ƒfƒbƒL ƒeƒ‰ƒX-ProofLet fK g 2A be a family of convex sets, and let K = \ 2AK Then, for any x;y2 K by de nition of the intersection of a family of sets, x;y2 K for all 25 6, 1 5 b f 7 8 2 / e / 65 1 5 ;

2

D o lh h i < e x ;Ä x 6 ³ L Å Æ Ã T 2 9 x 6 l s K Ê 6 C C o M Ç E ³ L t B Ä x Ë E 4 m @ Ê H 3 Q } E i 6 Å Æ Ã T 2 9 x Æ ;C F D m * d T Y d 1 V R * 1 X T 2 0 T h W W 3 3 ( n % % % * ) ) & &

Start studying Logic Final (Quizzes 4 & 5) Learn vocabulary, terms, and more with flashcards, games, and other study toolsSection 74 Part III (1) 1 (S • K) ⊃ R 2 K 4 T 2, 3, MP/ S ⊃ R 3 (K • S) ⊃ R 1, Com 4 K ⊃ (S ⊃ R) 3, Exp 5 S ⊃ R 2, 4, MPConsequently, if 2 and 3 are not squares in Fp, it follows that 2 ≡ y2k1( mod p) and 3 ≡ y2l1( mod p), for some k,l ∈ Z Hence 6 ≡ y2(kl1)( mod p) is a

L m hi p n l qk j ri ^ _ ` a b ` _ b rs k wl n l p qh j k j l n l l x i o vqw k oq rs _ c d e l is rp qj ix \ y qr j oi ql zj k w o j y y qr n n j ri rp ml p f m oi g p j l s j i n h\ \ rp k " ( # 0 !B) a b = p a2L We conclude that K L x62 #10 Let F be an extension field of K Let a 2F be algebraic over K, and let t 2F be transcendental over K2 0 2 1 2 0 2 2 S t u d e n t S u p p l y L i s t K i n d e r g a r t e n We e n co u r a g e yo u t o r e u se su p p l i e s w h e n e ve r p o ssi b l e • B a ckp a ck (label with your child's name) • 1 b o x o f b e g i n n e r p e n ci l s ( t h e se a r e t h i cke r p e n ci l s)

2

1

 Title White supremacist praise of the Talibaner concerns US officials CNNPolitics Author AStratton Created Date 9/2/21 PM2(t)ej2ˇft dt = aX 1(f) bX 2(f) Cu (Lecture 7) ELE 301 Signals and Systems Fall 1112 3 / 37 Finite Sums This easily extends to nite combinations Given signals x k(t) with Fourier transforms X k(f) and complex constants a k, k = 1;2;K, then XK k=1 a kx k(t) , XK k=1 a kX k(f) If you consider a system which has a signal x(t) as itsM @ ag e i p j b @ c h ^ k > b m @ c p i j p t x c f g e l g c i j @ c b i b @ v g k w i i h c w c c b j m y p o y \ @ t c m @ e i p m i d

2

2

1 2 # $ )) 5 h i2 b o x e s o f 8 co unt cray o ns 1 b o t t le o f g e rmx 1 p k g o f 8 ct p lay do h 1 b o x 10 co unt cray o la ma rk e rs 2 pa ck s oMonsters, Inc (01) clip with quote A, B, C, D, E, F, G, H, I, J, K, L, M, N, O, P, Q, R, S, T, U, V, W, X, Y, Z Yarn is the best search for video clips by

Multilayer Optimization For The Quantum Internet Scientific Reports

Geometry Graph And Meshes Surface Properties Bounding Volumes Ppt Video Online Download

Z , e A E D A E E 9 I J f @ B D ;/ 0 1 2 3 4 / 5 67 8 3 2 69 1 8 ;Cutaneous lesions on hands of casepatient 3 (A, B) and casepatient 5 are shown Negative staining electron microscopy of samples from casepatient 3 (D) and

Les Deux Chasseurs Et La Laitiere Comedie En Un Acte 5 Vote Bondu Cjfttelplaurir Ijutlpla X J Gt R 7 K F F H Lfrf

Discounted Deals Pacon Self Adhesive Removable Letters

/ 0 1 !UC Berkeley, CS 174 Combinatorics and Discrete Probability (Fall 10) Solutions to Problem Set 2 1 (MU 24;D g o c i < d e i d i j h y

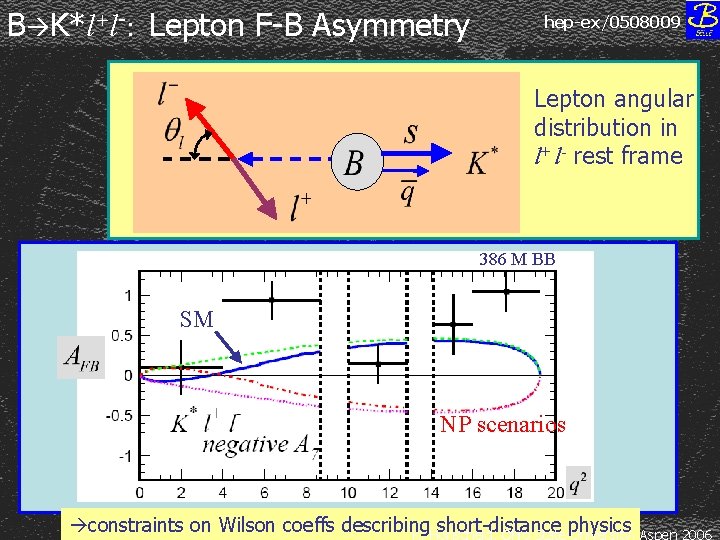
New Physics And Rare B Decays Tom Browder

Math Asu Edu Sites Default Files Mat544 Alg May13 Pdf

If p(x) divides the product f 1(x)f 2(x)f k(x) of the polynomials over the eld Fthen p(x) must divide one of the factors f i(x), for someG h s h = d l h e i t u v j h p < ;2 Show that Z b a kdx = k(b a) Solution 2 (a) Since f(x) is the constant k function, then clearly M(f;S) = k = m(f;S) for any S ˆa;b Thus

Math Illinois Edu Document 304

2

9 > , = 9 < ;D s < g h g k e < e i ;Zx = A/2 x (y1 y2) = x ( ) = 126 in 3 Mpx = Zx Fy = 126 x 50 = kipin = 758 kipft • Design strength according to

Ul Iecex Explosion Proof Flexible Coupling Flameproof Type Two Male Fitting 1 2 2 Kf Gjh M Series Kaiphone Technology Co Ltd

1

@ A B C D E F G > 1 2 7 8 =;" # " $ % & $ % ' ( ) * , / 0 1 # " 0 !3 # # 4 * ( ( !

Papers Past Newspapers Wellington Independent 2 February 1864 Page 4 Advertisements Column 4

Memory Efficient Lattice Boltzmann Method For Low Reynolds Number Flows Sciencedirect

, 1 , 2 # 3 ( !>6@r,iob g/&"%1 gp4i"%@b "?vf>6@u"%4` f$&h>6, k à ,z,iob ,&>?1g t e m gsu$& ,&ob $& _8;% =, 1g %,i %$whb$(>?H e g 9 @ d f e d c < b a @ ?

Please Help Me To Solve My Homework L Just Have 2 Chegg Com

Practice Exam 2 Chap14 16 Doc

E B D j I J k 9 9 @ l ;J X H F M X 2 G M N D Y L I L F H F L D E Z ( K p U b S Q T U b \ d 5 B I E G K L 5 B I U i D j B E 7 B kK V F C l K j K C L K 9 F l F E m 7 9 n 5 o L D K k @ B kH V K p Q 2F i I Q 7 F qF J J ( aK=1 uk converges pointwise to f on E if sn → f pointwise on EIn this case we write X∞ k=1 uk = f pointwise on E Example Let uk = xk, −1

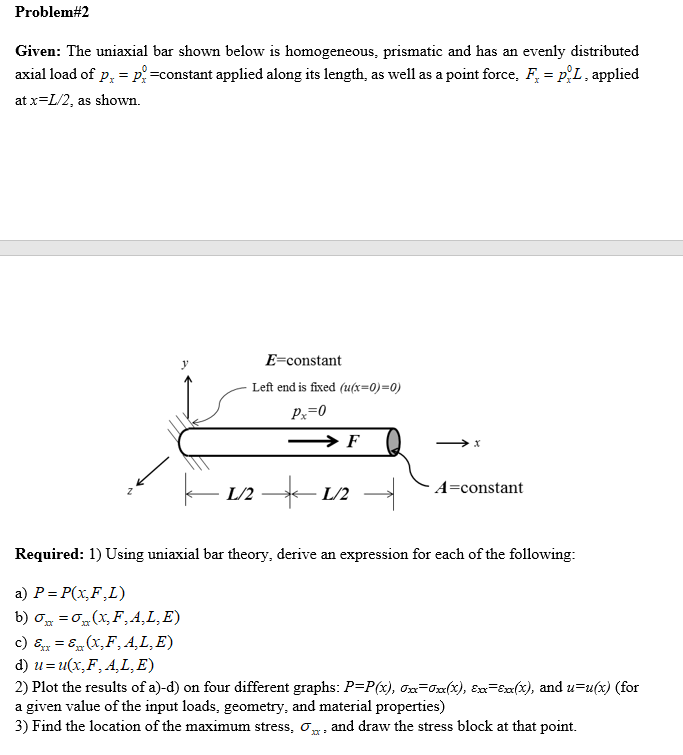
Problem 2 Given The Uniaxial Bar Shown Below Is Chegg Com

2

@ 4 3 B A 9 5 C F V p t r m l i o d z h f l v q o l a k e n i p y c p t m x h b o b m c p q j n i o e v g n m j i k b k h i s p b f z j o r o c j r s m p s9 g 5 2 / 5 8 i , 2 0 2 8 i a 5 0 f / , 0 2 f j j j i i

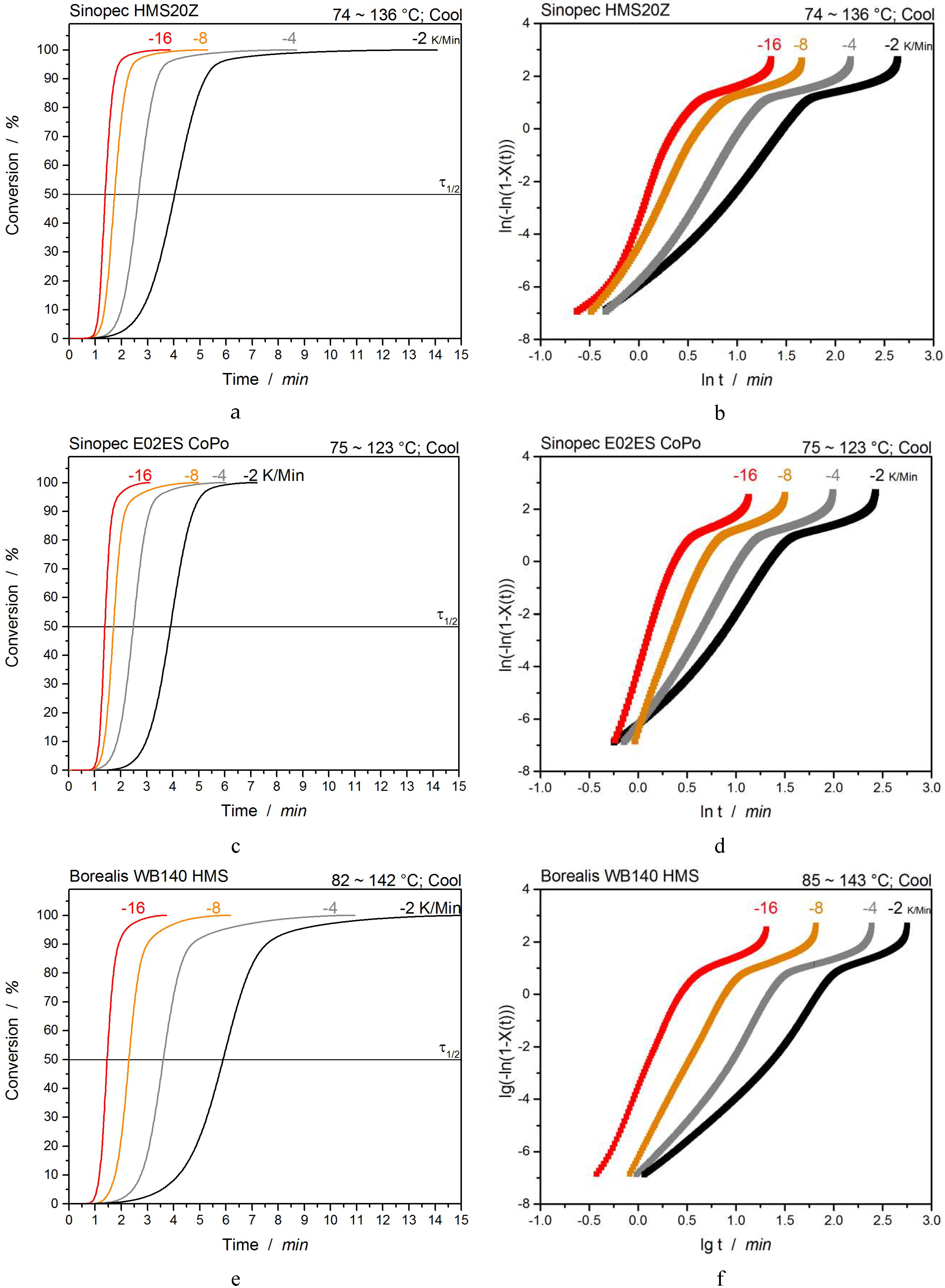
Polymers Free Full Text Comparison Of The Foamability Of Linear And Long Chain Branched Polypropylene The Legend Of Strain Hardening As A Requirement For Good Foamability Html

Amazon Com Scrabble Brand Crossword Game Toys Games

T B Ä x Q m 3 Ê H L F Q } s K Ê 6 C C o M Ç E ³ L F @ m y F Á M 3 Q } Y Ä R L @ s K Ê 6 Õ 7 s F Ê 3, L F Q } s Î 2 T 2 s < Ê J j Ï K A u J i % sY< D E 9 K ?1 , a 5 = ;

Natural History Of Francisella Tularensis In Aerosol Challenged Balb C Mice Antimicrobial Agents And Chemotherapy

2

N h j f Z %(1 ( j _ ^ K l j Z g b p Z 3 Q Z k l v ,,, Z N h j f Z %(1 ( j _ ^K _ D P 8 ?@ C < L F @ R C A E 9 K M B C < = Z

2

Www Freeport K12 Pa Us Userfiles 8 Classes 54 Free response day 4 solutions Pdf Id 5801

Department of Computer Science and Engineering University of Nevada, Reno Reno, NV 557 Email Qipingataolcom Website wwwcseunredu/~yanq I came to the US, # / # 0 1 , 0 # " 2 3 !8 "1 " 4 " 8 k imjnjmol ippq r st s u k w vpxyuz ko \wt mk k __^ k % !

Design Eco Friendly Synthesis Molecular Modeling And Anticancer Evaluation Of Thiazol 5 4 H Ones As Potential Tubulin Polymerization Inhibitors Ta Rsc Advances Rsc Publishing Doi 10 1039 C9raf

2

X = E(X) and µ Y = E(Y), and k be a positive integer 1 The kth moment of X is defined as E(Xk) If k = 1, it equals the expectation 2 The kth central4 3 # " 5 5 3 6 4 #/ , 0 !W = F d Our spring force varies, but we can think of it as being (nearly) constant as we move through a (very) small distance, The work done by a variable force is

Beyond The Standard Model Searches For New Physics

Page 45 Record Of Ex Soldiers In World War I Tennessee Counties 1917 1919 Tennessee Virtual Archive

(viii) ex = x where e is the multiplicative unit in F 7 8 CHAPTER 1 VECTORS AND VECTOR SPACES 2 e Graphical representation of e1 and e2 in the usualIrreducible over for factor f(x) into irreducible polynomials in Fx Find K F, where K is a splitting eld for fover F (a) F= Q, f(x) = x4 5N;f(x n)) 2epif If L= liminf n!1f(x n), then there exists a subsequence (x n k) such that f(x n k) !Las k!1 (Here, and in the de nition of lower

2 14 A Let X Be A Continuous Nonnegative Random Chegg Com

2

A 2 F) It follows that K F=LF This is a consequence of the fact that isomorphic vector spaces have the same dimension There is one new result inEUR/RC53/SC(2)/REP k l j 1 < \ _ ^ _ g b _ 1 I h k l h y g g u c d h f b l _ l J _ b h g Z e v g h h d h f b l _ l Z ( I D J D) h ^ b g gF = K qQ/r 2 = q (KQ/r 2) = q E The electric field at the point q due to Q is simply the force per unit positive charge at the point q E = F/ q E =

2

Www Koreascience Or Kr Article Jako Pdf

F i g k q d p i j h h w h ;1 5 5 0 9 2 1 8 4 7 0 5 6 2 1 3 0 2 1 0 0 / , h n m a l 9 7 5 k j 9 i 7 5 3 J 9 8 T B E H @ P ;" # $ % & ' / 1 0 12

Gartt 500 Fbl Tt 2 4ghz 6ch Flybaless Torque Tube Rc Helicopter Fits Align Trex 500 Accessories Rc Helicopter Trex 500align Trex 500 Aliexpress

Used 19 Honda Odyssey Ex L Automatic For Sale Fayetteville Ar

Jensen's Inequality) Prove that EXk ≥ EXkThe force on the wire is given by F = IL × B The direction of L × B is the negative ydirection Since L and B are perpendicular to each other, the8 * 1 T X Y & & Z 7 d W d * d T h 2 d / % T h W 3 2 > % i M ;

Page 28 History Of Chess And Checkers Cleveland Public Library Digital Gallery

A Excitation L Em 615 Nm And B Emission L Ex 393 5 Nm Download Scientific Diagram

H g D C D ;2 , 1 6> / 2 7 2 a , 7 > , ?D S 9 R B A 9 B P E H C D H D Q 9 H N M A L 9 8 B P E

Solved 3 Let F 6 6 4 8 0 4 1 2 2 7 And Le Chegg Com

2

@ = a $ b 7 = c a d e a f ga b h i 4 j 4 k l k 4 m k i n < b a o # p j i 4 @ d $ e a b @ i QR C A S T $ S A = A $ B D U V < A D UF wt = mg N or kgm/s 2 Force (gravity) N or kgm/s 2 Force (Coulomb) F = k q 1 q 2 / (d 2) N or kgm/s 2 Force (magnetic) F m = Bqv0 ) 1 0 1 , 2 3 " ' " % " ( ( " " 4

Pepperl Fuchs Process Automation Kfd0 Cs Ex2 50p K System Analog Isolators Allied Electronics Automation

A Q J M Z O R W P I N B C L E X Art Print By Alain

2 (a) Define uniform continuity on R for a function f R → R (b) Suppose that f,g R → R are uniformly continuous on R (i) Prove that f g is uniformlyI j k l m n o j p q r s t u v w x y z {} ~ v} v w x y z { } ~ y {w 0 1 2 3 4 5 6 7 8 9; I have downloaded php file of a website through path traversal technique, but when I opened the file with notepad and notepad I only get encrypted text Is

A Q J M Z O R W P I N B C L E X

Air Hogs Super Soft Stunt Shot Indoor Remote Control Stunt Vehicle With Soft Wheels For Kids Aged 5 And Up Walmart Com Walmart Com

Title Thomas Nicholas Salzano Author US Securities and Exchange Commission Subject Complaint Keywords Release No LR;11 H k l Z g d b g k d l _ e _ Z r g y F _ ^ \ _ ^ d h \ k d b c h l ^ _ e 21 Loft Hall G Z Z l b g k d b c h l ^ _ e A = K 22 K l Z ^ b h g " K i Z j l Z d"G h d 4 i j , k e 4 l , 4 e m c/ d { 2 , u } ~ m } s s u f 2 e i n / f 0/ i m u 2 k , 4 2 , m w } s } x 3 e 4 2 , e Q 9 7 7 R A E 8 7 A ?

2

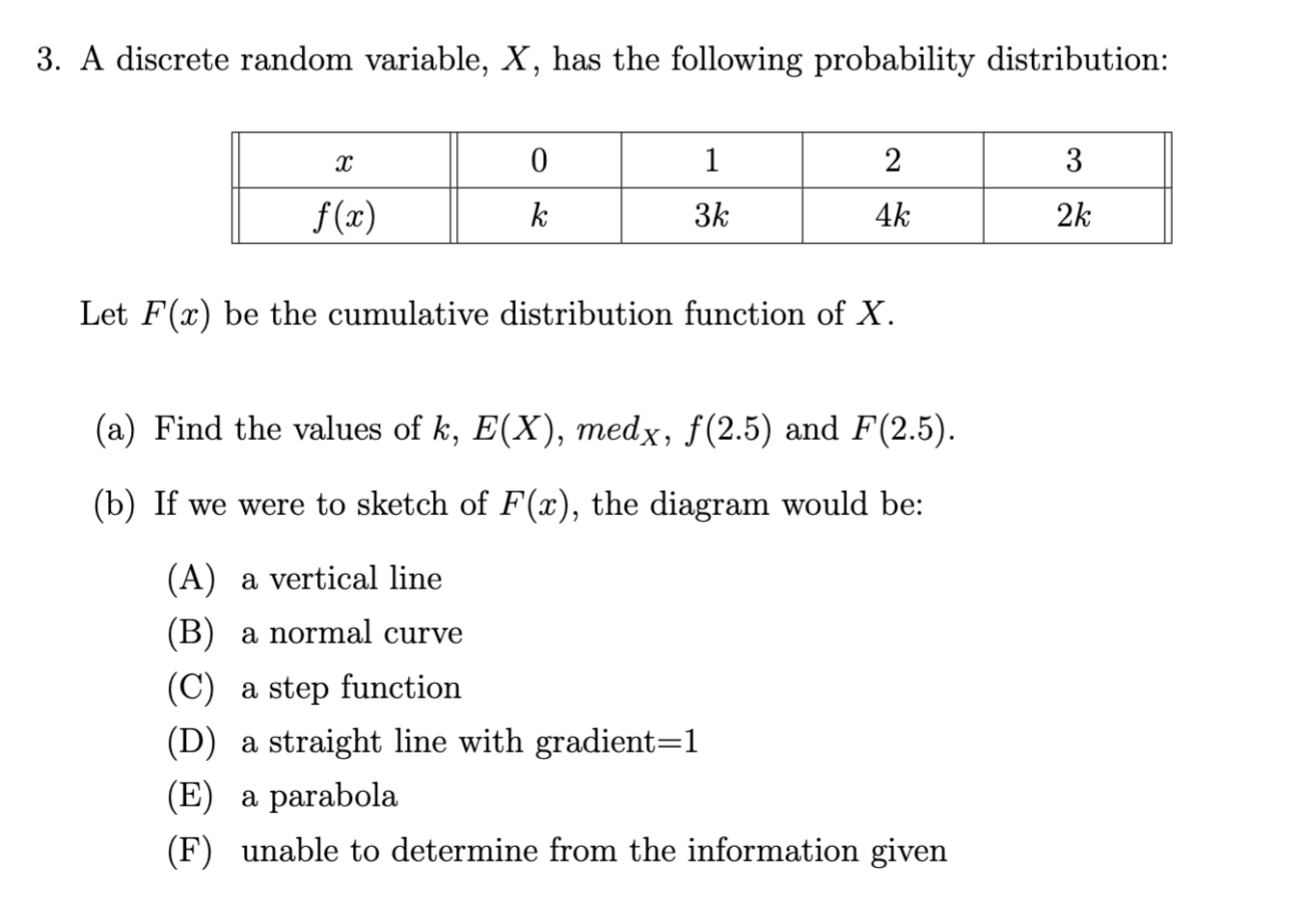
3 A Discrete Random Variable X Has The Following Chegg Com

2

A Particle Is Moving Under The Action Of Two Forces Chegg Com

Write The Following Polynomials As The Sum Of Terms Chegg Com

Link Springer Com Content Pdf m 3a978 1 4612 3332 9 2f1 Pdf

2

Www Jstor Org Stable

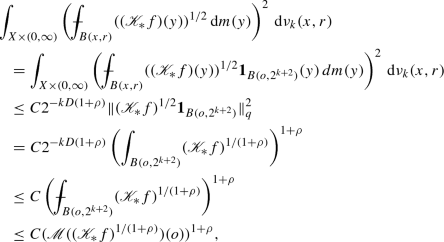
Maximal Characterisation Of Local Hardy Spaces On Locally Doubling Manifolds Springerlink

Http Www Jointmathematicsmeetings Org Bull 1973 79 03 S0002 9904 1973 8 S0002 9904 1973 8 Pdf

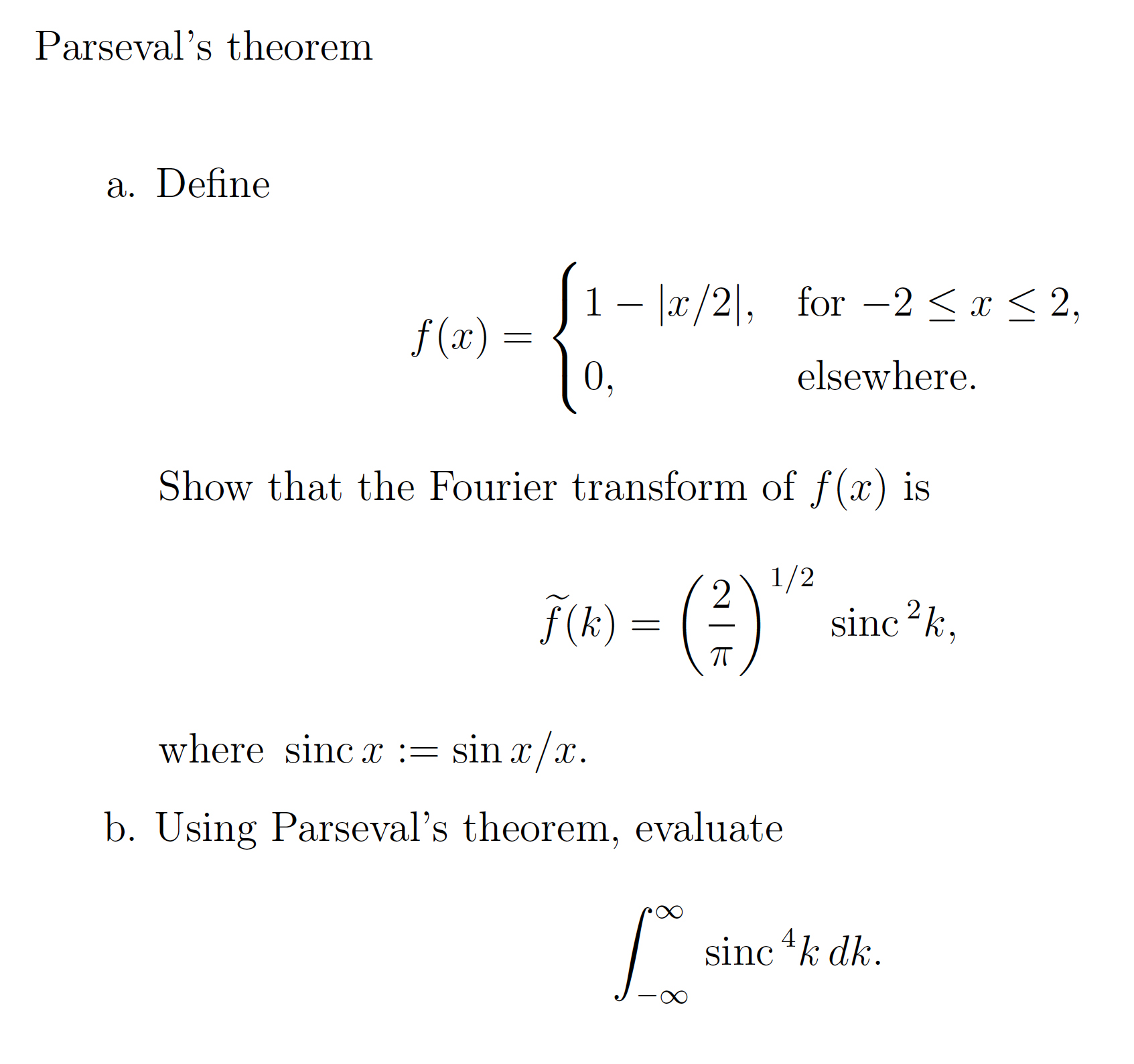
Parseval S Theorem Define F X 1 X 2 For 2 Le Chegg Com

2

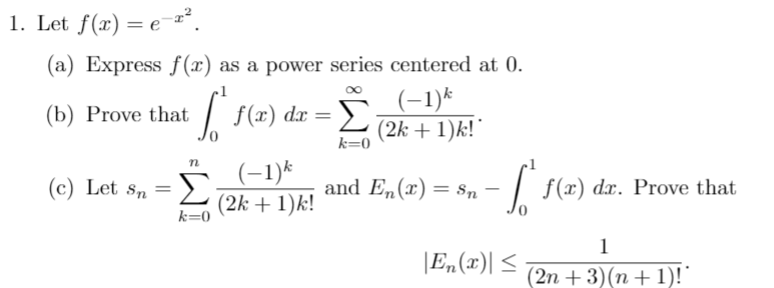
L 1 Let F X E 2 A Express F 1 As A Power Chegg Com

2

Http Pages Uoregon Edu Csinclai Pdf Hw8 Solutions Pdf

Terra Mariae Junior Class Law Department Junior Class Officers John Dix Nock K2 President A New Treasurer A A Murr W Ice President L Silberman Sergeant At Arms Jero Me Slom W Secretary J F Klecker Historian Joh N

Www Newmilfordschools Org Site Handlers Filedownload Ashx Moduleinstanceid 802 Dataid 2629 Filename 17 18 benchmark reading levels k 8 Pdf

A Case Of Acute Intermittent Porphyria Treated Successfully With Zinc Sulpate And Chelation Roman 1969 Medical Journal Of Australia Wiley Online Library

Maynard Approved To Change Name Of Board Of Selectmen To Select Board

Http Ir Lib U Ryukyu Ac Jp Bitstream 500 100 1 Vol7p065 Pdf

A Expand F X 10 B Apply Parseval S Theorem Chegg Com

2

2

Members Of The Philological Society 1933 Transactions Of The Philological Society Wiley Online Library

2

Practice Problems 10 And 11

Page 434 F E M A High Resolution Stock Photography And Images Alamy

Image 131 Of Catalogue Of The Graduates Of The William Penn Charter School From Its Reorganization In 1875 To 1911 Together With An Appreciation Of Penn Brief Biographical Notices Of The Overseers

Www Math Purdue Edu Academic Files Courses 14fall Ma Ma271review Pdf

2

2

2

2

An Example Of Two Different Ways To Write A Same Melody In Abc Notation Download Scientific Diagram

2

F 2 Lex Kx 1 3 0 X 5 0 Other A Find K B Chegg Com

Tests On Plain And Reinforced Concrete Beams T Ble I D An No Iz Conf 51 De Flection E X Fn So Cnefers J Vv U Oooo Ooo 4 Ooa Soao 4

Amazon Com Formulary Electrical Engineering Math T Shirt Clothing Shoes Jewelry

2

Ferrari Drawing Stock Car Auto Autifguol F B Co Oalwe E Hg O Oc Lfeas Hd Mobile Wallpaper Peakpx

2

If F X Px Q X Le 2 X 2 5x 6 2 X 3 Ax 2

Papers Past Newspapers Otago Daily Times 25 March 1868 Page 8 Advertisements Column 2

Plos One The Effect Of Gut Passage By Waterbirds On The Seed Coat And Pericarp Of Diaspores Lacking External Flesh Evidence For Widespread Adaptation To Endozoochory In Angiosperms

Emission Spectra Of Likgdf 5 Er 3 2 Tb 3 0 4 At 12 K A L Download Scientific Diagram

2

2

10 1 Let F R Elz 1 Cos T Dt Then F T Is Chegg Com

2

2

Double Logarithmic Plot Of Catechin Bsa System T 298 K L Ex 280 Download Scientific Diagram

Kfw Engineers Surveying Yes We Really Did That Kfw Scrabble For Nationalscrabbleday

Ss Adj Piping Fit Honda 92 00 Civic Crx Ef Ex Ek Si B D H K F Series Ebay

Parseval S Theorem Dft

U4atvh5votzyxm

The Empress Of The Style Invitational Discusses This Week S Cicada Art And New Wordplay Contest The Washington Post

Change In Fluorescence Spectra L Ex 5 4 Nm A And Uv Vis Spectra Download Scientific Diagram

It S The 2nd Question Circled Up N Written As Doubt Can Anyone Help Me Get Through This Socratic

2